The Golden Mean - Golden Ratio - Fibonacci

Golden Ratio

The golden ratio (symbol is the Greek  letter "phi" shown at left) is a special number approximately equal  1.618 It appears many times in geometry, art,  architecture and other  areas. The Golden ratio is a special number found by  dividing a line into two  parts so that the longer part divided by the  smaller part is also equal  to the  whole length divided by the longer part.  It is often symbolized using  phi,  after the 21st letter of the Greek alphabet.  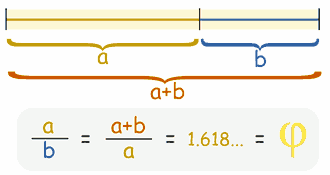In an equation form, it looks  like this a/b = (a+b)/a = 1.6180339887498948420 … We find the golden ratio when we divide  line into  two parts so that: the longer part divided  by  the smaller  part is also equal to the whole length divided  by  the longer  part As with pi (the ratio of the circumference of  a circle to its diameter),  the digits go  on and on, theoretically into infinity.  Phi is usually rounded off to 1.618.  This number has been discovered and rediscovered many times, which is why it has so many names — the Golden mean,  the Golden section, divine proportion, etc.  Historically, the number can be seen in the architecture of many ancient creations, like the Great Pyramids and the Parthenon.  In the Great Pyramid of Giza, the length  of each side of the base is 756 feet with a height of 481 feet.  The ratio of the base to the height is roughly 1.5717, which is close to the Golden ratio. Around 1200, mathematician Leonardo Fibonacci discovered the  unique properties  of the Fibonacci sequence. This sequence ties directly into the  Golden ratio  because if you take any two successive Fibonacci numbers,  their ratio is very  close to the Golden ratio. As the numbers get higher, the ratio  becomes even closer  to 1.618. For example, the ratio of 3 to 5 is 1.666.  Getting even higher, the ratio of 144 to 233 is 1.618. But the ratio of 13 to 21 is 1.625.   These numbers are all successive  numbers in the Fibonacci sequence. Many buildings and artworks have  the Golden Ratio in them,  such as the Parthenon in Greece,  but it is not really known  if it was designed that way. These numbers can be applied to the  proportions of a rectangle,  called the Golden  rectangle. This is known as one of the  most visually satisfying of  all geometric  forms – hence, the appearance of the  Golden ratio in art.  The Golden rectangle is also  related to the Golden spiral, which is  created by making adjacent  squares of Fibonacci dimensions. In 1509, Luca Pacioli wrote a book that  refers to the number as the "Divine Proportion,"  which was illustrated by Leonardo da Vinci.  Da Vinci later called this sectio aurea or  the Golden section. The Golden ratio was  used to achieve balance  and beauty in many  Renaissance paintings and sculptures.  Da Vinci himself used  the Golden ratio to define  all of the proportions in his Last Supper,  including the dimensions  of the table and the  proportions of the walls and backgrounds.  The Golden ratio also  appears in  da Vinci's Vitruvian Man and the Mona Lisa.  Other artists who employed the  Golden ratio include Michelangelo, Raphael,  Rembrandt, Seurat, and Salvador Dali. Flower petals: The number of petals on  some flowers follows the Fibonacci  sequence. It is believed that in the Darwinian processes,  each petal is placed to allow for the best possible exposure to sunlight and other factors. Seed heads: The seeds of a flower are  often produced at the center and migrate outward to fill  the space. For example, sunflowers  follow this pattern. Pinecones: The spiral pattern of the seed  pods spiral upward in opposite  directions. The number of steps the spirals take tend  to match Fibonacci numbers. Tree branches: The way tree branches form  or split is an example of the  Fibonacci sequence. Root systems and algae  exhibit this formation pattern. Shells: Many shells, including snail shells and nautilus shells, are perfect  examples of the  Golden spiral. Spiral galaxies: The Milky Way has a number of spiral arms, each of which has a logarithmic spiral of roughly 12 degrees. The shape of the spiral is identical to the Golden  spiral, and the  Golden rectangle can be drawn over any spiral galaxy. Hurricanes: Much like shells, hurricanes often display the Golden spiral. Fingers: The length of our fingers, each section from the tip of the base to  the wrist is larger than the preceding one by roughly the ratio of phi. Animal bodies: The measurement of the human navel to the floor and the top  of the head to the  navel is the Golden ratio. But we are not the only examples of the Golden ratio in the animal  kingdom; dolphins, starfish, sand dollars, sea urchins, ants and honeybees  also exhibit the  proportion. DNA molecules: A DNA molecule measures 34 angstroms by 21 angstroms  at each full cycle of the double helix spiral. In the Fibonacci series, 34 and 21 are successive numbers. NOW HERE IS AN GOLDEN MEAN IN SOME OF OUR MOST POPULAR BRANDS .... 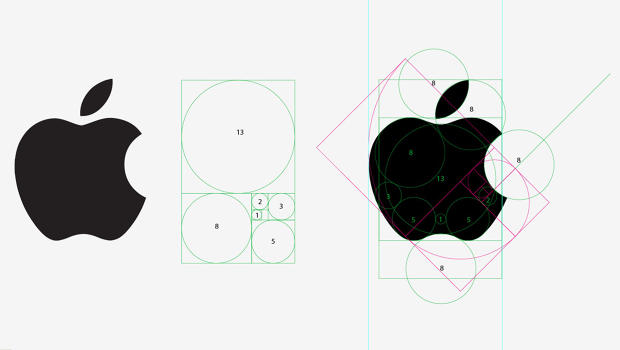if you enjoyed this blog on "The Golden Ratio" please read my blog about "Holograms" HOLOGRAMS : HOW DO THEY WORK   this is the link. enjoy